§24 Steady simple waves

Let us consider now the two dimensional problem of steady plane parallel flow at infinity which turns through an angle as it flows round a curved profile. A particular case of this problem occurs when the flow turns through an angle (Landau & Lifshitz, 1995). For this particular situation the Prandtl-Meyer flow is obviously the solution and so, the hydrodynamical quantities depend on a single variable, the angle $\phi$ measured from a defined axis at the onset of the curvature. Because of this, all quantities can be expressed as functions of each other. Since this case is a particular solution to the general problem, it is natural to seek the solutions of the equations of motion in which the quantities $p, n, \ensuremath{v}_x, \ensuremath{v}_y$ can be expressed as a function of each other. Evidently this imposes a restriction on the solution of the equations of motion since for two dimensional flow, any quantity depends on two coordinates, $x$ and $y$, and so any chosen hydrodynamical variable can be written as a function of any other two.

Because of the fact that the flow is uniform at infinity, where all quantities are constant, particularly the entropy, and because the flow is steady, the entropy is constant along a streamline. Thus, if there are no shock waves in the flow, the entropy remains constant along the whole trajectory of the flow and in what follows we will use this result.

In this case, Euler's equation, eq.(9.7), and the continuity equation, eq.(9.1), are respectively:

$$\ensuremath{v}_x \frac{ \partial \ensuremath{v}_x }{ \partial x }... ... \ensuremath{\mathsf{c}}^2 }{ \gamma \omega } \frac{ \partial p }{ \partial x},$$

$$\ensuremath{v}_x \frac{ \partial \ensuremath{v}_y }{ \partial x }... ... \ensuremath{\mathsf{c}}^2 }{ \gamma \omega } \frac{ \partial p }{ \partial y},$$

$$\frac{ \partial }{ \partial x } \left( \gamma \ensuremath{v}_x n ... ...+ \frac{ \partial }{ \partial y } \left( \gamma \ensuremath{v}_y n \right) = 0.$$

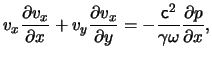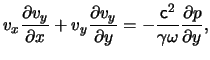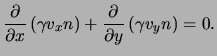Rewriting these equations as Jacobians we obtain:

$$\ensuremath{v}_x \frac{ \partial ( \ensuremath{v}_x, y ) }{ \part... ...athsf{c}}^2 }{ \gamma \omega } \frac{ \partial ( p, y ) }{ \partial ( x, y ) },$$

$$\ensuremath{v}_x \frac{ \partial ( \ensuremath{v}_y, y ) }{ \part... ...athsf{c}}^2 }{ \gamma \omega } \frac{ \partial ( p, x ) }{ \partial ( x, y ) },$$

$$\frac{ \partial ( \gamma \ensuremath{v}_x n, y ) }{ \partial ( x,... ... - \frac{ \partial ( \gamma \ensuremath{v}_y n, x ) }{ \partial ( x, y ) } = 0.$$

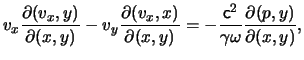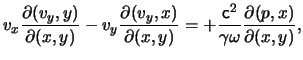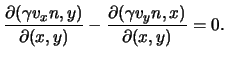We now take the coordinate $x$ and the pressure $p$ as independent variables. To make this transformation we have to multiply the previous set of equations by $\partial ( x, y ) / \partial ( x, p )$. This multiplication leaves the equations the same, but with the substitution $\partial ( x, y ) \! \rightarrow \partial ( x, p )$. Expanding this last relation and because all quantities are now functions of the pressure $p$ but not of x, it follows that:

$$\left( \ensuremath{v}_y - \ensuremath{v}_x \frac{ \partial y }{ \... ...\ensuremath{\mathsf{c}}^2 }{ \gamma \omega } \frac{ \partial y }{ \partial x },$$

$$\left( \ensuremath{v}_y - \ensuremath{v}_x \frac{ \partial y }{ \... ...\ensuremath{\mathsf{c}}^2 }{ \gamma \omega } \frac{ \partial y }{ \partial x },$$

$$\left( \ensuremath{v}_y - \ensuremath{v}_x \frac{ \partial y }{ \... ...\partial x } \frac{ \mathrm{d} \ensuremath{v}_x }{ \mathrm{d} p } \right\} = 0.$$

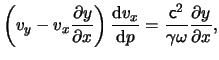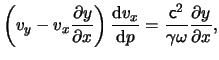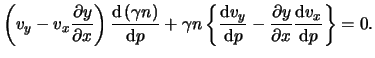Here we have taken $\partial y / \partial x$ to mean the derivative at constant pressure: $( \partial y / \partial x )_p$. Since every hydrodynamic quantity is assumed to be a function of the pressure, then in the previous set of equations it necessarily follows that $\partial y / \partial x$ is a function which depends only on the pressure, that is $( \partial y / \partial x )_p = f_1(p)$. Therefore:

$$y = x f_1(p) + f_2(p).$$ (24.1)

No further calculations are needed if we use the solution for the case in which a rarefaction wave is formed when flow turns around an angle (Landau & Lifshitz, 1995). This solution is given by the results of section §23. As was mentioned in that section, all hydrodynamical quantities are constant along the characteristic lines $\phi \! = \! \textrm{const}$. This particular solution of the flow past an angle obviously corresponds to the case in which $f_2(p) \! = 0$ in eq.(24.1). The function $f_1(p)$ is determined from the equations obtained in section §23.

For a given constant value of the pressure $p$, eq.(24.1), gives a set of straight lines in the $x$   -$y$ plane. These lines intersect the streamlines at the Mach angle. This is due to the fact that the lines $y \! = \! x f_1(p)$ for the particular solution of the flow through an angle have this property. In other words, one family of characteristic surfaces correspond to a set of straight lines along which all quantities remain constant. However, for the general case, this lines are no longer concurrent.

The properties of the flow as described above are analogous to the non-relativistic equivalent known as simple waves (Landau & Lifshitz, 1995). In what follows we will use this name to refer to such a flow.

Figure IV.2: Supersonic uniform flow at the left of the diagram bends around a curved profile OH. The Mach angle $\alpha_1$ is the angle made by the characteristics and the streamlines before the onset of the curvature. The characteristics make an angle $\phi_1$ with the ``continuation'' of the rarefaction wave formed at the onset of the curvature and the angle $\phi$ is measured from the line 0A'. The curvature causes the characteristic lines to intersect eventually and this occurs at point K in the diagram, giving rise to a shock wave represented as the segment KL.

Let us now construct the solution for a simple wave once a fixed profile is given. Consider the profile as shown in fig.(IV.1). Plane parallel steady flow streams from the left of the point O and flows around the given profile. Since we assume that the flow is supersonic, the effect of the curvature starting at O is communicated to the flow only downstream of the characteristic OA generated at point O. The characteristics to the left of OA, region 1, are all parallel and intersect the $x$ axis at the Mach Angle $\alpha_1$ given by eq.(11.20):

$$\sin \alpha_1 = \frac{ \sqrt{ 1 - \left( \ensuremath{v}_1 / \ensu... ...eft( a / \ensuremath{\mathsf{c}}\right)^2 } } \frac{ a }{ \ensuremath{v}_1 },$$ (24.2)

where the velocity $\ensuremath{v}_1$ is the velocity of the flow to the left of the characteristic OA. In eqs.(23.11)-(23.18) the angle $\phi$ of the characteristics is measured with respect to some straight line in the $x$   -$y$ plane. As a result, we can choose for those equations the constant of integration $\phi_0 \equiv 0 \!$This means that the line from which the angle $\phi$ is measured has been chosen in a very particular way. In order to find the line which is the characteristic for $\phi \! = \! 0$, let us proceed as follows. When $\phi \! = \! 0$ and the gas is ultrarelativistic, eqs.(23.13)-(23.14) show that the velocity $\ensuremath{v}\! = \! a$ and for the classical case, it follows from eqs.(23.17)-(23.18) that the velocity takes the value $\ensuremath{v}\! = \! c$. In both cases this means that the line $\phi \! = 0$ corresponds to the point at which the flow has reached the value of the local velocity of sound. This, however, is not possible since we are assuming that the flow is supersonic everywhere. Nevertheless, if the rarefaction wave is assumed to extend formally into the region to the left of OA, we can use these relations and then the characteristic line must correspond to a value of $\phi$ given by:

$$\phi_1 = \sqrt{ \frac{ \kappa + 1 }{ \kappa - 1 } } \arccos \left( \frac{ c_1 }{ c_* } \right),$$ (24.3)
for a classical gas according to eq.(23.15), and

$$\phi_1 = \frac{ \ensuremath{\mathsf{c}}}{ a } \arccos \frac{ \sqr... ...ensuremath{\mathsf{c}})^2 } }{ \sqrt{ 1 - ( a / \ensuremath{\mathsf{c}})^2 } },$$ (24.4)

for the ultrarelativistic case according to eq.(23.7), eq.(23.10) and eq.(23.12). The angle between the characteristics and the $x$ axis is then given by: $\phi_* - \phi$, where $\phi_* \! = \alpha_1 + \phi_1,$    and the angle $\alpha_1$ is the Mach angle in region 1. The $x$    and $y$ velocity components in terms of the azimuthal angle $\theta$ are given by:

$$\ensuremath{v}_x = \ensuremath{v}\cos \theta, \qquad \ensuremath{v}_y = \ensuremath{v}\sin \theta,$$ (24.5)

and the values for the magnitude of the velocity, the angle $\theta$ and the pressure are given by:

$$\ensuremath{v}^2 = c_*^2 \left\{ 1 + \frac{ 2 }{ \kappa - 1 } \sin^2 \sqrt{ \frac{ \kappa - 1 }{ \kappa + 1 } } \phi \right\},$$ (24.6)

$$\begin{split}\theta & = \phi_* - \phi - \alpha, \ & = \phi_*... ...rac{ \kappa - 1 }{ \kappa + 1 } } \phi \right\}, \ \end{split}$$ (24.7)

$$p = p_* \cos^{ 2 \kappa / \left( \kappa - 1 \right) } \sqrt{ \frac{ \kappa - 1 }{ \kappa + 1 } } \phi ,$$ (24.8)

for a classical gas according to eqs.(23.15)-(23.18) and using the fact that the Poisson adiabatic for a polytropic gas means that: $p c^{- 2 \kappa / \left( \kappa - 1 \right) } \! = \! \textrm{const}$. In the case of an ultrarelativistic gas, eqs.(23.12)-(23.14) together with Bernoulli's equation and the fact that the enthalpy density $\omega \! = \! \kappa p / \left( \kappa - 1 \right)$ give:

$$\ensuremath{v}^2 = \ensuremath{\mathsf{c}}^2 \left\{ 1 - \left( 2 - \kappa \right) \cos^2 \sqrt{ \kappa - 1 } \phi \right\},$$ (24.9)

$$\begin{split}\theta & = \phi_* - \phi - \alpha, \ & = \phi_*... ...ppa -1 } \cot \sqrt{ \kappa - 1 } \phi \right\}, \ \end{split}$$ (24.10)

$$p = p_0 \left( 2 - \kappa \right)^{ - \kappa / 2 \left( \kappa -1... ...ght) } \cos^{ - \kappa / \left( \kappa - 1 \right) } \sqrt{ \kappa - 1 } \phi .$$ (24.11)

Since the angle $\phi_* - \phi$ is the angle between the characteristics and the $x$ axis, it follows that the line describing the characteristics is:

$$y = x \tan \left( \phi_* - \phi \right) + G(\phi).$$ (24.12)

The function $G(\phi)$ is obtained from the following arguments for a given profile of the curvature (Landau & Lifshitz, 1995). If the equation describing the shape of the profile is given by the points $X$    and $Y$ where $Y \! = \! Y(X)$, the velocity of the gas is tangential to this surface, and so:

$$\tan \theta = \frac{ \mathrm{d} Y }{ \mathrm{d} X }.$$ (24.13)

Now, the equation of the line through the point $(X,Y)$ which makes an angle $\phi_* - \phi$ with the $x$ axis is:

$$y - Y = \left( x - X \right) \tan \left( \phi_* - \phi \right).$$ (24.14)

Eq.(24.14) is the same as eq.(24.12) if we set:

$$G(\phi) = Y - X \tan ( \phi_* - \phi ).$$ (24.15)

If we start from a given profile $Y \! = \! Y(X)$ then, using eq.(24.13) we can find the parametric set of equations: $X \! = \! X(\theta)$    and $Y \! = \! Y(\theta)$. Substitution of $\theta \! = \! \theta(\phi)$ from eq.(24.7) or eq.(24.10) depending of whether the gas is classical or ultrarelativistic, we find $X \! = \! X(\phi)$    and $Y \! = \! Y(\phi)$. Substitution of this in eq.(24.15) gives the required function $G(\phi)$.

If the shape of the surface around which the gas flows around is convex, the angle $\theta$ that the velocity vector makes with the $x$ axis decreases downstream. The angle $\phi - \phi_*$ between the characteristics leaving the surface and the $x$ axis also decreases monotonically. In other words, characteristics for this kind of flow do not intersect and we form a continuous and rarefied flow.

On the other hand, if the shape of the surface is concave as shown in fig.(IV.2), the angle $\theta$ increases monotonically and so does the angle the characteristics make with the $x$ axis. This means that there must exist a region in the flow in which characteristics intersect. The value of the hydrodynamical quantities is constant for every characteristic line. This constant however changes for different non-parallel characteristics. In other words, at the point of intersection different hydrodynamical quantities -for example, the pressure- are multivalued. This situation can not occur and results in the formation of a shock wave. This shock wave cannot be calculated from the above considerations, since they were based on the assumption that the flow had no discontinuities at all -the entropy was assumed to be constant. However, the point at which the shock wave starts, that is point K in fig.(IV.2), can be calculated from the following considerations. We can work out the inclination of the characteristics $\phi$ as a function of the coordinates $x$    and $y$. This function $\phi(x,y)$ becomes multivalued when these coordinates exceed certain fixed values, say $x_0$    and $y_0$. At a fixed $x \! = \! x_0$ the curve giving the value of $\phi$ as a function of $y$ becomes multivalued. That is, the derivative $\left( \partial \phi / \partial y \right)_x \! = \! \infty$, or $\left( \partial y / \partial \phi \right)_x \! = \! 0$. It is evident that at the point $y \! = \! y_0$ the curve $\phi(y)$ must lie in both sides of the vertical tangent, else the function $\phi(y)$ would already be multivalued. This means that the point $(x_0,y_0)$ cannot be a maximum, or a minimum of the function $\phi(y)$ but it has to be an inflection point. In other words, the coordinates of point K in fig.(IV.2) can be calculated from the set of equations (Landau & Lifshitz, 1995):

$$\left( \frac{ \partial y }{ \partial \phi } \right)_x = 0, \qquad \quad \left( \frac{ \partial^2 y }{ \partial \phi^2 } \right)_x = 0.$$ (24.16)

When the profile is concave, the streamlines that pass above the point O in fig.(IV.2) pass through a shock wave and the simple wave no longer exist. Streamlines that pass below this point seem to be safe from destruction. However, the perturbing effect from the shock wave KL influences this region also, and so it is not possible to describe the flow there as a simple wave. Nevertheless, since the flow is supersonic, the perturbing effect of the shock wave is only communicated downstream. This means that the region to the left of the characteristic PK (which corresponds to the other set of characteristics emanating from point P) does not notice the presence of the shock wave. In other words, the solution mentioned above, in which a simple wave is formed around a concave profile is only valid to the left of the segment PKL.

Footnotes

... Jacobians

The Jacobian $\partial ( a, b ) / \partial ( x, y )$ is defined as:

$$\frac{ \partial (a, b) }{ \partial (x,y) } = \textrm{det} \begin... ...rtial y \\ \partial b / \partial x & \partial b / \partial y \end{bmatrix},$$

It is obvious that $\partial ( a, y ) / \partial ( x, y ) \! = \! \partial a / \partial x,$    and that $\partial ( a, b ) / \partial ( x, y ) \! = \! - \partial ( b, a ) / \partial ( x, y )$