§23 Prandtl-Meyer flow

Let us describe briefly the exact solution of the equations of hydrodynamics for plane steady flow depending on one angular variable $\phi$ only. This problem was first investigated by Prandtl and Meyer in 1908 (Courant & Friedrichs, 1976; Landau & Lifshitz, 1995) for the case in which relativistic effects were not taken into account. The full relativistic solution to the problem is due to Kolosnitsyn & Stanyukovich (1984).

For this case, Euler's equation, eq.(9.6) and the continuity equation, eq.(9.1) can be written:

$$\ensuremath{v}_\phi = \frac{ \mathrm{d} \ensuremath{v}_r }{ \mathrm{d} \phi },$$ (23.1)

$$\ensuremath{v}_r n \gamma + \frac{ \mathrm{d} \left( \ensuremath{v}_\phi n \gamma \right) }{ \mathrm{d} \phi } = 0,$$ (23.2)

$$\omega \gamma / n = ,$$ (23.3)

where $\ensuremath{v}_r$    and $\ensuremath{v}_\phi$ are the components of the velocity in the radial and azimuthal directions respectively. Eq.(23.3) is the Bernoulli equation, eq.(9.8), for this problem.

Using the definition of the speed of sound, eq.(11.3), in eq.(23.2) with the aid of eq.(23.3) it is found that:

$$\ensuremath{v}_r + \frac{ \mathrm{d} \ensuremath{v}_\phi }{ \math... ...a^2 } \frac{ \mathrm{d} }{ \mathrm{d} \phi } \ln \left( \omega / n \right) = 0.$$ (23.4)

On the other hand, differentiation of $\gamma^{-2}$ with respect to the azimuthal angle $\phi$ and using eq.(23.3), gives:

$$\ensuremath{v}_\phi \left( \ensuremath{v}_r + \frac{ \mathrm{d} \... ...ight) \frac{ \mathrm{d} }{ \mathrm{d} \phi } \ln \left( \omega / n \right) = 0.$$ (23.5)

Multiplication of eq.(23.4) by $\ensuremath{v}_\phi$ and substracting this from eq.(23.5) gives:

$$\ensuremath{v}_\phi^2 = a^2 \left( 1 - \frac{ \ensuremath{v}_r^2 }{ \ensuremath{\mathsf{c}}^2 } \right).$$ (23.6)

On the other hand, Bernoulli's equation, eq.(23.3), together with the value of the specific enthalpy for a polytropic gas given in eq.(12.4), can be rewritten

$$\left( 1 - \frac{ \ensuremath{v}_r^2 + \ensuremath{v}_\phi^2 }{ \... ... \frac{ 1 }{ \kappa -1 } \frac{ a_0^2 }{ \ensuremath{\mathsf{c}}^2 } \right)^2,$$ (23.7)

in which it has been assumed that at some definite point, the flow velocity vanishes and the speed of sound has a value $a_0$ there. It is always possible to make the velocity zero at a certain point by a suitable choice of the system of reference.

Eqs.(23.6)-(23.7) can be solved in terms of $\ensuremath{v}_r$    and $\ensuremath{v}_\phi$:

$$\ensuremath{v}_r^2 / \ensuremath{\mathsf{c}}^2 = 1 - F^2(a),$$ (23.8)

$$\ensuremath{v}_\phi^2 = a^2 F^2(a),$$ (23.9)
where

$$F^2(a) = \left( 1 - \frac{ 1 }{ \kappa - 1 } \frac{ a_0^2 }{ \ens... ...frac{ 1 }{ \kappa - 1 } \frac{ a^2 }{ \ensuremath{\mathsf{c}}^2 } \right)^{-2}.$$ (23.10)

Because $\ensuremath{v}_r \mathrm{d} \ensuremath{v}_r = \ensuremath{\mathsf{c}}^2 F(a) F'(a) \mathrm{d} a$, eq.(23.1) gives the required solution (Kolosnitsyn & Stanyukovich, 1984):

$$\phi + \phi_0 = \pm \ensuremath{\mathsf{c}}\int{ \frac{ F'(a) \mathrm{d} a }{ a \sqrt{ 1 - F^2(a) } } }.$$ (23.11)

This equation gives the speed of sound as a function of the azimuthal angle. From eqs.(23.8)-(23.9) it follows that the radial and azimuthal velocities can be obtained as a function of the same angle $\phi$. Because of this, all the remaining hydrodynamical variables can be found. The sign in eq.(23.11) can be chosen to be negative by measuring the angle $\phi$ in the appropriate direction and we will do that in what follows.

Let us consider now the case of an ultrarelativistic gas and integrate eq.(23.11) by parts, to obtain:

$$\phi + \phi_0 = \frac{ \ensuremath{\mathsf{c}}}{ a } \arccos F(a) + \ensuremath{\mathsf{c}}\int{ \frac{ \mathrm{d} a }{ a^2 } \arccos F(a) }.$$ (23.12)

For the case of an ultrarelativistic gas, the speed of sound $a$ is given by eq.(12.6). In other words, this velocity is constant and so the integral in eq.(23.12) is a Lebesgue integral. Since this integral is taken over a bounded and measurable function over a set of measure zero, its value is zero.

Using eqs.(23.8)-(23.9) and eq.(23.12) the desired solution is obtained (Kolosnitsyn & Stanyukovich, 1984; Königl, 1980):

$$\ensuremath{v}_r = \ensuremath{\mathsf{c}}\sin \left\{ \sqrt{ \kappa - 1 } \left( \phi + \phi_0 \right) \right\},$$ (23.13)

$$\ensuremath{v}_\phi = \sqrt{ \kappa - 1 } \ensuremath{\mathsf{c}}\cos \left\{ \sqrt{ \kappa - 1 } \left( \phi + \phi_0 \right) \right\},$$ (23.14)

for an ultrarelativistic equation of state of the gas.

For the classical case, in which $\ensuremath{\mathsf{c}}\! \rightarrow \! \infty$, eq.(23.11) gives for a polytropic gas with polytropic index $\kappa$:

$$\phi + \phi_0 = - \sqrt{ \frac{ \kappa + 1 }{ \kappa - 1 } }\int{ \frac{ \mathrm{d} \zeta }{ \sqrt{ 1 - \zeta^2 } } },$$
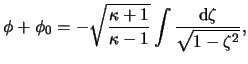where

$$\zeta \equiv \frac{ a }{ \ensuremath{\mathsf{c}}} \sqrt{ \frac{ \... ...ppa -1 } \frac{ a_0^2 }{ \ensuremath{\mathsf{c}}^2 } \right)^2 \right\}^{-1/2},$$
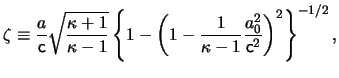and so, the required solution is (Kolosnitsyn & Stanyukovich, 1984):

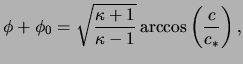$$\phi + \phi_0 = \sqrt{ \frac{ \kappa + 1 }{ \kappa - 1 } } \arccos \left( \frac{ c }{ c_* } \right),$$ (23.15)

where the speed of sound $a$ has been rewritten as $c$ to be consistent in the non-relativistic case. The critical velocity of sound $c_*$ is given by (Landau & Lifshitz, 1995):

$$c_*^2 = \frac{ 2 }{ \kappa + 1 } c_0^2.$$ (23.16)

The value for the velocities can thus be calculated from eq.(23.1) and eq.(23.9) with $F(a) \! = \! 1$:

$$\ensuremath{v}_r = \sqrt{ \frac{ \kappa + 1 }{ \kappa - 1 } } c_* \sin \sqrt{ \frac{ \kappa - 1 }{ \kappa +1 } } \left( \phi - \phi_0 \right),$$ (23.17)

$$\ensuremath{v}_\phi = c = c_* \cos \sqrt{ \frac{ \kappa - 1 }{ \kappa +1 } } \left( \phi - \phi_0 \right).$$ (23.18)

Some important inequalities must be satisfied for the flow under consideration. First of all, eq.(23.11) together with eq.(12.4) and the first law of thermodynamics imply that $\mathrm{d} p / \mathrm{d} \phi \! < \! 0$. Using this inequality and the fact that $\mathrm{d} e \! = \! \ensuremath{\mathsf{c}}^2 \mathrm{d} p / a^2$ combined with the first law of thermodynamics, it follows that $\mathrm{d} n / \mathrm{d} \phi \! < \! 0$. Also, using eqs.(23.8)-(23.9) it follows that $\mathrm{d} \ensuremath{v} / \mathrm{d} \phi \propto - \mathrm{d} a / \mathrm{d} \phi$ and necessarily $\mathrm{d} \ensuremath{v}/ \mathrm{d} \phi \! > \! 0$.

On the other hand, the angle $\chi$ that the velocity vector makes with a definite axis is related to the velocity and the azimuthal angle $\phi$ by:

$$\chi = \phi + \arctan \left( \ensuremath{v}_\phi / \ensuremath{v}_r \right)$$ (23.19)

as it is seen from fig.(IV.1). Thus, since the $\phi$ component of Euler's equation, eq.(9.6) implies that:

$$\left( \ensuremath{v}_r + \frac{ \partial \ensuremath{v}_\phi }{ ... ...th{\mathsf{c}}^2 \frac{ \partial \left( \omega / n \right) }{ \partial n} = 0,$$

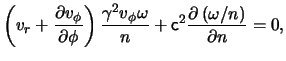
it follows that $\mathrm{d} \chi / \mathrm{d} \phi \! = - \left( \ensuremath{v}^2 \gamma^2 \omega / \ensuremath{\mathsf{c}}^2 \right)^{-1} \mathrm{d} p / \mathrm{d} \phi$.

Figure IV.1: Relation between the velocity vector $\boldsymbol{ \ensuremath{v}} \! = \! \ensuremath{v}_r \hat{ \boldsymbol{e} }_r + \ensuremath{v}_\phi \hat{ \boldsymbol{e} }_\phi$ and the angle $\chi$, as a function of the azimuthal angle $\phi$. $\chi$ is the angle that the velocity vector makes with certain fixed axis with origin O.

In other words, we have proved that for the flow for which we are concerned, the following inequalities are satisfied:

$$\mathrm{d} p / \mathrm{d} \phi < 0, \quad \mathrm{d} n / \mathrm{... ...nsuremath{v}/ \mathrm{d} \phi > 0, \quad \mathrm{d} \chi / \mathrm{d} \phi > 0.$$ (23.20)

A flow with these properties is often described as a rarefaction wave (Landau & Lifshitz, 1995) and we will use this name in what follows.

Another, very important property of this rarefaction wave is that the lines at constant $\phi$ intersect the streamlines at the Mach angle, that is, they are characteristics. Indeed, from fig.(IV.1), it follows that the angle $\alpha$ between the line $\phi \! = \! \textrm{const}$ and the velocity vector $\boldsymbol{\ensuremath{v}}$ is given by $\sin \alpha = \ensuremath{v}_\phi / \ensuremath{v}$. Using eqs.(23.8)-(23.10) it follows that this relation can be written as eq.(11.20). Because all quantities in the problem are functions of a single variable, the angle $\phi$, it follows that every hydrodynamical quantity is constant along the characteristics.